Elastodynamics of point defects revisited

Elastizitätstheorie, Punktdefekt, Feldtheorie, lokale Eichfeldtheorie, QFT, QED

Idea: This chapter's result provides an equation of motion for point defects in an elastic medium, and is in principle independent from the rest of the work. Yet this equation of motion is used in its four-dimensional form in the next chapter – and interpreted as an equation of motion for leptons. This application provides an interpretation for multiple quantum mechanical constants.

 

In words

Point defects are local deformations in an elastic lattice.
In this chapter a complete description will be worked out for the treatment of a moving point defect in an elastic lattice. The established model (e.g. in ) will be expanded with the help of modern mathematics, especially using considerations on local symmetry. The model of a defect at rest in a lattice will be used as a starting point, and this model will be expanded to include the defect’s interaction with the neighbouring lattice, its motion and the effects which arise when including a defect into a lattice. In the end the case of partially disordered lattice structures will be discussed.

A brief overview of the individual sections:

 

Description of a defect in the lattice

The point defect will be described as a harmonic oscillator with a slightly different force constant and therefore slightly different oscillations than the rest of the oscillators in the lattice.

The approach is the following:

1. Step: the elastic lattice is described without a defect, the model only contains point masses which are coupled through springs.
2. Step: the system is decoupled by a mathematical operation, such that the lattice can be described as a collection of independent harmonic oscillators.
3. Step: now an isotropic point defect is modelled by varying slightly the force constant of a single oscillator. Hereby the coupling of the overall system is renewed.

Schematic illustration of the approach to including a defect into the lattice:

 

Interaction of the defect with lattice oscillations

At first, the oscillation field of the defect and the one of the unperturbed environment can be described separately, since the defect causing the renewed coupling is small. Then the interaction is introduced as a perturbative term which describes the coupling of the defect to the rest of the lattice. A few assumptions about the interaction can be made when considering the spherical symmetry of the problem. Due to the nature of oscillations, only the parts of the oscillation having a symmetry complying with the one of the defect contribute to the interaction. All other parts cancel each other out in the sum and do not have to be considered any further. As a result, the problem is greatly simplified.

 

Motion

Schematic illustration of the vacancy model and of the potential (from ).

Schematic illustration of the averaged potential.

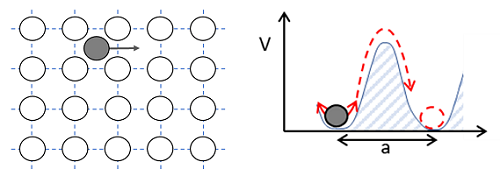
Defects wander and move in real mediums. By adding a motion-term one can describe the influence of the defects’ movement to its deflection field. Similar to streamlines in fluid mechanics, the description will use a momentaneous picture of the field, as opposed to the conventional approach for describing defect dynamics, where it is tried to parametrise the path in time (this would correspond to the description of pathlines in fluid mechanics).

Since the defect can wander by many lattice positions from its original position, the harmonic oscillator approximation is not sufficient to model the movement. Instead the vacancy model for diffusion (read in ) is adapted to the set of equations reached so far. In this model it is assumed that a defect can jump from one lattice gap to another and when doing this it crosses a symmetrical potential (See Figure on the top right).

If the wandering of the defect occurs on many lattice positions, the potential can be averaged. The corresponding potential is developed and introduced (lower Figure on the right).

 

Effects from intrinsic generation of the defect

Defects can be added externally into the medium. Thermodynamically they often also appear in an intrinsic manner in the medium. The most famous intrinsic defect in real crystals is the Frenkel defect : An atom fills a non prescribed position, simultaneously leaving a regular lattice position empty.

Schematic illustration of a Frenkel-defect:
Point mass in the wrong place, thereby creating a vacancy.

 

Description of multiple defects

When describing multiple defects at different places in the lattice, it no longer suffices to require global symmetry, one must rather require the symmetry to hold separately at each defect position. Together with the statements above this results in a complete theory of the interaction, which corresponds to a local gauge field.

 

Symmetry breaking

The lattice locally breaks the isotropy of space.

One can view this as a phase transition: in a liquid, particles slide randomly past each other. Thus, on average, they produce an isotropic structure. When reaching the phase transition (e.g. by cooling) to a solid state, the motion becomes ever stiffer and is replaced by rigid bounds. An ordered, crystalline structure is formed in the process, which is not isotropic anymore. The symmetry of the isotropy was broken in this phase transition.

When cooling faster, partially ordered (amorphous) structures are also possible, as seen e.g. in glass formations. At long range, i.e. averaged over many elementary cells, one can still find isotropy in such amorphous structures, since the lattice is randomised to a certain degree. The breaking of symmetry is then only local.

Phase transitions can be represented mathematically with a parametrised, additional potential. The parameter is then a measure for how far into the transition the medium is advanced, or analogously, by which degree the symmetry is broken.

Schematic transition from isotropic structures to ordered crystalline structures:

With the formation of the crystalline structure and the symmetry breaking, some typical effects arise, which can be observed in elastic lattices with finite lattice constants. In particular the effect of the localised oscillation modes: Around the defects, the lattice possesses slightly different properties than its environment, which leads to slightly different eigenfrequencies. Hence, oscillation modes are allowed, which can move around the defect but not in the rest of the lattice. These oscillations are exponentially suppressed and are called local oscillation modes in the classical theory. In the symmetry breaking description this corresponds to massive oscillation modes.  

Mathematically

Lagrangian of the unperturbed lattice

If one considers the model of an elastic lattice in three (and four) dimensions with forces only between direct neighbours, a single point mass at equilibrium position [math]\displaystyle{ \mathbf{m} }[/math] can be described by the following Lagrangian:

<equation id="eqn:su3.01" shownumber> [math]\displaystyle{ \displaystyle L_\mathbf{m}=T_\mathbf{m}-V_\mathbf{m} = \frac{M \, {\mathbf{\dot{u}}^2_\mathbf{m}}}{2} - \left[\Phi_0 + \frac{1}{2}\sum_{neighb. \, \mathbf{n}} \mathbf{\Phi_{mn}}\left(\mathbf{u_m-u_n}\right)^2 + o(\mathbf{u}^3)\right] }[/math] </equation>

[math]\displaystyle{ \mathbf{u_m} }[/math] describes the deflection of the point mass from the equilibrium position [math]\displaystyle{ \mathbf{m} }[/math]. [math]\displaystyle{ \mathbf{n} }[/math] is the sum of the neighbouring positions with point masses which interact with [math]\displaystyle{ \mathbf{m} }[/math]. [math]\displaystyle{ M }[/math] describes the mass of a single point mass, [math]\displaystyle{ \Phi_0 }[/math] a base potential – if required – and [math]\displaystyle{ \mathbf{\Phi_{mn}} }[/math] the strength of the harmonic forces between the point masses at Positions [math]\displaystyle{ \mathbf{m} }[/math] and [math]\displaystyle{ \mathbf{n} }[/math]. Terms of higher order are neglected.

The Lagrangian of the whole lattice can be represented as the sum of the single Lagrangians of each point mass:

<equation id="eqn:su3.01" shownumber> [math]\displaystyle{ \displaystyle L=\sum_\mathbf{m} L_\mathbf{m} }[/math] </equation>

Remarks:

• One assumes that the functions behave well. Then, in the continuum limit, [math]\displaystyle{ L_\mathbf{m} }[/math] turns into the Lagrange density [math]\displaystyle{ \mathcal{L} }[/math] of a deflection field.
• This system of equations gives solutions for the defelctions [math]\displaystyle{ \mathbf{u_m} }[/math] up to a constant normalizing factor: if [math]\displaystyle{ \mathbf{u_m} }[/math] is a solution, then [math]\displaystyle{ \mathbf{u^\prime_m}=k \cdot \mathbf{u_m} }[/math] also solves the corresponding Euler Lagrange system of equations.

The Lagrangian of a single point is now varied a bit:

 

Change of coordinates

The Lagrangian is rewritten in more convenient coordinates [math]\displaystyle{ \mathbf{v}=\sqrt M \mathbf{u} }[/math]. This gives:

<equation id="eqn:su3.01" shownumber> [math]\displaystyle{ \displaystyle L_\mathbf{m}= \frac{{\mathbf{\dot{v}}^2_\mathbf{m}}}{2} - \left[\frac{\Phi_0}{M} + \frac{1}{2}\sum_{neighb. \, \mathbf{n}} \frac{\mathbf{\Phi_{mn}}}{M}(\mathbf{v_m-v_n})^2 + o(\mathbf{u}^3)\right] }[/math] </equation>

 

Static case

In the static case one can remove the kinetic term:

<equation id="eqn:su3.01" shownumber> [math]\displaystyle{ \displaystyle L_\mathbf{m}= -V_\mathbf{m} = - \frac{\Phi_0}{M} - \frac{1}{2}\sum_{neighb. \, \mathbf{n}} \frac{\mathbf{\Phi_{mn}}}{M}(\mathbf{v_m-v_n})^2 - o(\mathbf{u}^3) }[/math] </equation>

 

Tensor notation

The square of the distances can be seen as derivatives and the spring constant is normalised on the microscopic lattice (written with a tilde). In the most general case, all directional derivatives contribute to the Lagrangian, so tensor notation is helpful:

<equation id="eqn:su3.01" shownumber> [math]\displaystyle{ \displaystyle L_m=-\frac{{\Phi{}}_0}{M}\ -\frac{a^2{\Phi{}}_{ij}}{2M}\ {\partial{}}_iv_j{\partial{}}^iv^j\ -o\left(\mathbf{v}^3\right)\ \ =-{\overset{\sim}{\Phi{}}}_0\ -\frac{1}{2}{\overset{\sim}{\Phi{}}}_{ij}\ {\partial{}}_iv_j{\partial{}}^iv^j\ -o\left(\mathbf{v}^3\right)\ \ =-{\overset{\sim}{\Phi{}}}_0\ -\frac{1}{2}{\overset{\sim}{\Phi{}}}_{ij}\ V_{ij}V^{ij} }[/math] </equation>

Where [math]\displaystyle{ a }[/math] is the distance between two point masses at equilibrium in the lattice and [math]\displaystyle{ \partial_{m_j-n_j}v_j \approx \frac{(v_{m_j}-v_{n_j})}{a} }[/math] are the components, here written as [math]\displaystyle{ \partial_i v_j \approx \frac{(v_{m_j}-v_{m_j-i})}{a} }[/math], where [math]\displaystyle{ i=m_j-n_j }[/math] is the [math]\displaystyle{ j }[/math]-th component of the distance between the two point masses at positions [math]\displaystyle{ \mathbf{m} }[/math] and [math]\displaystyle{ \mathbf{n} }[/math].

 

Decoupling of the oscillators

Since it is a harmonic system, the single oscillators can be decoupled with an appropriate coordinate transformation:

<equation id="eqn:su3.01" shownumber> [math]\displaystyle{ \displaystyle L_m=\ -{\overset{\sim}{\Phi{}}}_0\ -\frac{1}{2}{\mathbf{\overset{\sim}{\Phi}_m}}\ {\left(\mathbf{w_m}\right)}^2 -o\left(\mathbf{w}^3\right) }[/math] </equation>

Subsequently one gets a set of equations for individual, independent oscillators.

Yet this decoupling is only possible in the unperturbed case and it is broken if there are defects. In the case of an isotropic lattice, even the directional derivative disappears, leaving:

<equation id="eqn:su3.01" shownumber> [math]\displaystyle{ \displaystyle L_m=\ -{\overset{\sim}{\Phi{}}}_0\ -\frac{1}{2}{{\overset{\sim}{\Phi}}}\ {\left(\mathbf{w_m}\right)}^2 -o\left(\mathbf{w}^3\right) }[/math] </equation>

 

Isotropic point defects

Introduction of a small, isotropic defect

The starting point is a decoupled, elastic lattice. A single, isotropic point defect can now be added by changing one single spring constant. This will be achieved by starting from the spring constant of the unperturbed lattice and varying it by a very small value step by step.

The change will break the decoupling of the other oscillators step by step. It is eligible to assume that the resulting coupling can be treated as a small perturbation of the Lagrangian. I.e. the Lagrangian of the overall system can be separated in a Lagrangian for the defect, a Lagrangian for the lattice and a term which describes the interaction/coupling between these two:

<equation id="eqn:su3.01" shownumber> [math]\displaystyle{ L_\mathbf{m}=L_{\mathbf{m},\ defect}+L_{\mathbf{m},\ lattice}+L_{\mathbf{m},\ inter.\ def.\leftrightarrow{}lattice} }[/math] </equation>

The single terms will now be looked at in more detail:

 

Lagrangian of a defect

The Lagrangian of the defect at the position [math]\displaystyle{ {\alpha} }[/math] can be written as follows according to the construction:

<equation id="eqn:su3.01" shownumber> [math]\displaystyle{ \displaystyle L_{\mathbf{m=\alpha},\ defect}=-V=-\frac{\overset{\sim}{\Phi}\xi}{2}\ {\left(\mathbf{w_\alpha}\right)}^2 }[/math] </equation>

Together with the spring constant of an unperturbed lattice this gives a harmonic oscillator with a slightly varied spring constant [math]\displaystyle{ {\overset{\sim}{\Phi}}^\prime=\overset{\sim}{\Phi}(1+\xi) }[/math], where [math]\displaystyle{ \xi=\frac{{\overset{\sim}{\Phi}}^\prime-\overset{\sim}{\Phi}}{\overset{\sim}{\Phi}} }[/math] is the relative strength of the defect, if the deflection field through the defect is normalised to [math]\displaystyle{ \int{\mathbf{w_\alpha^\dagger w_\alpha}}\ dV=1 }[/math]. In the following the relative Lagrangian will be used:

<equation id="eqn:su3.01" shownumber> [math]\displaystyle{ \displaystyle L_{\mathbf{m=\alpha},\ defect,rel.}=-V=-\frac{\xi}{2}\ {\left(\mathbf{w_\alpha}\right)}^2 }[/math] </equation>

Where the normalisation is taken into account with adjusted spring constants (the relative spring constant is marked with a double tilde). For any spring constant [math]\displaystyle{ \overset{\sim}{X} }[/math]:

<equation id="eqn:su3.01" shownumber> [math]\displaystyle{ \displaystyle \overset{\approx}{X}=\frac{\overset{\sim}{X}}{\overset{\sim}{\Phi{}}} }[/math] </equation>

 

Lagrangian of the lattice

Since in the general case it cannot be decoupled anymore, the Lagrangian of the lattice takes the following basic form:

<equation id="eqn:su3.01" shownumber> [math]\displaystyle{ L_{\mathbf{m},\ lattice}=-\overset{\approx}{\Phi}_0\ -{\overset{\approx}{\Phi}}_{ij}\ V_{ij}V^{ij} }[/math] </equation>

Here it is assumed that the dual (conjugate complex) fields are independent of each other, whereby the initial factor ½ is removed such that the equations of motion remain unchanged.

With the help of symmetry considerations one can make assumptions on the inner structure of the components which interact with the defect:

• Only those components of the oscillation which have the same symmetry as the defect will contribute to interactions. All other components cancel each other out through summation.
• The defect is isotropic and its Lagrangian is proportional to the square of the deformation, so it has both a phase and a spherical symmetry.
• The local symmetry of the Lagrangian must be arbitrary such that the description of defects works for any position and especially for any configurations with multiple defects.
• Since its components are isotropic, the force constant looses its directional dependency, which significantly simplifies the problem. Furthermore one can take the bulk modulus (e.g. ) of the lattice to be the force constant. So altogether the force constant is:

<equation id="eqn:su3.01" shownumber> [math]\displaystyle{ {\overset{\approx}{\Phi}}_{ij}=\overset{\approx}{\Phi}=\overset{\approx}{K} }[/math] </equation>

These considerations require that the Lagrangian of the lattice at rest be symmetric (invariant) with respect to local transformations of the symmetry group [math]\displaystyle{ U(1) }[/math] for the phase and [math]\displaystyle{ SO(3) }[/math] for the isotropy in three dimensions, so altogether [math]\displaystyle{ U(1)\times SO(3) }[/math]. (In four dimensions the relevant symmetry groups are [math]\displaystyle{ U(1)\times SU(2) }[/math]).

The general Lagrangian can be divided into parts which correspond to the demanded symmetries:

<equation id="eqn:su3.01" shownumber> [math]\displaystyle{ \displaystyle L_{m,\ lattice}=-{\overset{\approx}{\Phi}}_0\ -{\left[\overset{\approx}{K}\cdot V_{ij}V^{ij}\right]}_{U(1)}-{\left[\sum_{a=1,2,3}\overset{\approx}{K}\cdot{V^a}_{ij}{V^a}^{ij}\right]}_{SO(3)} }[/math] </equation>

 

Lagrangian of the interaction – static

Oscillations and movement are the source of interaction in an elastic lattice. So the interaction term disappears in the static case:

<equation id="eqn:su3.01" shownumber> [math]\displaystyle{ L_{\mathbf{m},\ inter.\ def.\leftrightarrow{}lattice}=0 }[/math] </equation>

Remarks on the symmetry:
In the static case both the Lagrangian of the lattice and the Lagrangian of the defect fulfil the symmetry conditions. The Lagrangian of the overall system is therefore also invariant with respect to these symmetry operations.

Directional movement of the defect necessarily destroys this symmetry. Because of conservation of momentum the interaction must therefore be just as strong that the original symmetry is reinstated.

With this idea in mind, the movement of the defect will be modelled. This model will then be built on to construct the interaction term in the dynamical case.  

Movement of the defect

Within the model of an elastic lattice, no big movement is possible for the defect. Yet in real materials, it is normal to have defects moving through diffusion. Since in an elastic lattice the (spring) bonds must be broken for this to happen, the model isn’t appropriate for describing the diffusion mechanism. A more adequate description is given by the vacancy model, where the potentials are represented by balls. A single ball can move from a lattice gap to another by passing in between two other balls, while crossing a symmetric potential (e.g. , read in ):

If the lattice constant becomes very small and the movement bears over multiple lattice positions, the potential can be averaged and assumed to be constant:

Schematic illustration of the vacancy model and of the bypassed potential (from ).

Schematic illustration of the averaged potential.

Since it is a directional movement the force constant which is necessary for the movement is given by the longitudinal modulus [math]\displaystyle{ \overset{\approx}{M} }[/math] of the elastic medium.

The movement from gap to gap can be described more easily by shifting the deflections by one position, i.e. the deflection at the position [math]\displaystyle{ \mathbf{m} }[/math] is substituted by the deflection from a neighbouring position [math]\displaystyle{ \mathbf{n} }[/math]:

<equation id="eqn:su3.01" shownumber> [math]\displaystyle{ \mathbf{w_m^\prime}=\mathbf{w_m} + (\mathbf{w_n} - \mathbf{w_m}) }[/math] </equation>

This transformation can be understood as a discrete derivative, which is substituted by the corresponding directional derivative in the transition to the constant potential:

<equation id="eqn:su3.01" shownumber> [math]\displaystyle{ \mathbf{w_m^\prime}=\mathbf{w_m}+\left(\mathbf{w_n}-\mathbf{w_m}\right) \approx \mathbf{w_m}-a \, \mathbf{∂}_{(\mathbf{m-n})}\mathbf{w_m} =\left(1-a\cdot 1\cdot \mathbf{∂} \right)\mathbf{w_m} }[/math] </equation>

With the change in the deflection field:

<equation id="eqn:su3.01" shownumber> [math]\displaystyle{ \mathbf{dw_m}=\mathbf{w_m^\prime}-\mathbf{w_m} = -a \cdot 1\cdot \mathbf{∂ w_m} }[/math] </equation>

In the Lagrangian the movement acts on the square of the deflection, which can be expanded analogously:

<equation id="eqn:su3.01" shownumber> [math]\displaystyle{ {\left(\mathbf{w_m^{\dagger}w_m}\right)}^\prime = \left(\mathbf{w_m^{\dagger} w_m}\right) - a\left(\mathbf{{∂}_{(m-n)}w_m^{\dagger}}\right)\mathbf{w_m} - a\ \mathbf{w_m^{\dagger}}\left(\mathbf{{∂}_{(m-n)}w_m}\right)+… \approx \mathbf{w_m^{\dagger}}\left(1-a\cdot 1\cdot \mathbf{\overleftrightarrow{∂}}\right)\mathbf{w_m} }[/math] </equation>

Where the derivative marked with the double arrow acts once on the right side and once on the left side.

By rewriting this, it yields the square of the change of the deflection field:

<equation id="eqn:su3.01" shownumber> [math]\displaystyle{ \mathbf{d}\left(\mathbf{{w_m}^2}\right)={\left(\mathbf{w_m^{\dagger}w_m}\right)}^\prime-\left(\mathbf{w_m^{\dagger}w_m}\right) =-\mathbf{w_m^{\dagger}}\left(a\cdot 1\cdot \mathbf{\overleftrightarrow{∂}}\right)\mathbf{w_m} }[/math] </equation>

In general the complete movement is given by the generator of the movement times the longitudinal modulus. The generators of the movement are operators of the form [math]\displaystyle{ \mathbf{M\cdot ∂} }[/math], where [math]\displaystyle{ \mathbf{∂} }[/math] is a directional derivative and [math]\displaystyle{ \mathbf{M} }[/math] a set of matrices which fulfil the following properties:

1. Each dimension has a linearly independent operator.
2. They are normalised and therefore length conserving.
3. They have real eigenvalues (=Hermitian), such that the momentum is observable.

In the basic case of a single defect moving in space, the matrix [math]\displaystyle{ \mathbf{M} }[/math] can be substituted by a scalar([math]\displaystyle{ \mathbf{M}=1 }[/math]) and the derivative can be written as the directional derivative [math]\displaystyle{ \mathbf{∂}=\left({\partial_x,\partial_y,\partial_z}\right) }[/math] .

In summary, the Lagrangian can be written as:

<equation id="eqn:su3.01" shownumber> [math]\displaystyle{ \displaystyle L_{\mathbf{m},\ defect,rel.} = \mathbf{w_\alpha^\dagger} \left(-\frac{\xi}{2}+\overset{\approx}{M}\cdot 1\cdot \mathbf{\overleftrightarrow{∂}}\right) \mathbf{w_\alpha} }[/math] </equation>

This Lagrangian can be somewhat simplified if [math]\displaystyle{ \mathbf{w_\alpha^\dagger} }[/math] and [math]\displaystyle{ \mathbf{w_\alpha} }[/math] are assumed to be independent fields. The alternative Lagrangian

<equation id="eqn:su3.01" shownumber> [math]\displaystyle{ \displaystyle L_{\mathbf{m},\ defect,rel.} = \mathbf{w_\alpha^\dagger} \left(-\xi+2\overset{\approx}{M}\cdot 1\cdot \mathbf{\overleftrightarrow{∂}}\right) \mathbf{w_\alpha} }[/math] </equation>

leads to the same equations of motion if it is assumed that the deflection field and its conjugate complex counterpart are independent from each other, and is a bit more convenient to work with.

 

Lagrangian of the interaction – dynamic

The directional movement of the defect destroys the symmetry of the static Lagrangian. Because of conservation of momentum, the interaction must be just strong enough as to reinstate the original symmetry. The overall Lagrangian remains thus invariant under the original symmetry operations.

<equation id="eqn:su3.01" shownumber> [math]\displaystyle{ \displaystyle L_{\mathbf{m},\ inter.\ def.\leftrightarrow{}lattice} = -\mathbf{w_\alpha}^\dagger \left({\left[3\overset{\approx}{K}\cdot M^j v_j\right]}_{U(1)} +{\left[\sum_{a=1,2,3} 3\overset{\approx}{K}\cdot M^j{R_a v^a}_j\right]}_{SO(3)}\right)\mathbf{w_\alpha} }[/math] </equation>

With [math]\displaystyle{ M^j }[/math], the components of the generators of the the movement and [math]\displaystyle{ R_a }[/math] the generator of the group [math]\displaystyle{ SO(3) }[/math] (respectively [math]\displaystyle{ SU(2) }[/math] in four dimensions).  

 

Intrinsic defects

Frenkel-Defekt (schematic):
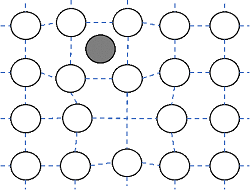
Point mass at the wrong location, thereby simultaneously creating a gap.

With the introduction of a foreign defect into the medium, its energy and mass are automatically increased. This energy corresponds to the base energy of the defect.

If the defect emerges intrinsically, i.e. solely from the inside of the medium, at least the mass must be conserved. For example, with the erroneous positioning of a point mass, a non prescribed position is filled and a gap is created at another position (Frenkel defect):

The interesting part here is the energy conservation: Without considering the static effects, when introducing a Frenkel defect in a relaxed, elastic lattice, energy must be expended on both sides, since originally all mass points are in the energetically most convenient position. In a set of equations where both sides of the defect are treated simultaneously, the base energy is:

<equation id="eqn:su3.01" shownumber> [math]\displaystyle{ \displaystyle L_{\mathbf{m},\ defect,rel.} = -\mathbf{W_{\alpha}^\dagger} \left(\left(\begin{array}{cc} 1 & 0 \\ 0 & 1 \end{array}\right) \xi \right) \mathbf{W_\alpha} }[/math] </equation>

Where the deflection function [math]\displaystyle{ \mathbf{U_\alpha} }[/math] was extended such that it can be split into the two parts of the defect (gap and additional mass), each of which correspond to a three dimensional deflection field:

<equation id="eqn:su3.01" shownumber> [math]\displaystyle{ \mathbf{W_\alpha}=\left(\begin{array}{cc} \mathbf{w_1} \\ \mathbf{w_2} \end{array}\right) }[/math] </equation>

In a medium which is originally not in a state of equilibrium but in a uniformly tense state, one side of the defect causes a release of energy, the other side expends energy. In an ideal model one can assume the total energy of the system to be conserved:

<equation id="eqn:su3.01" shownumber> [math]\displaystyle{ L_{\mathbf{m},\ defect,rel.} = \mathbf{\overline{W}_\alpha}\xi{}\mathbf{W_\alpha} \quad }[/math] where [math]\displaystyle{ \quad \mathbf{\overline{W}_\alpha} = \mathbf{W_\alpha^\dagger} \left(\begin{array}{cc} 1 & 0 \\ 0 & -1 \end{array}\right) }[/math] as well as [math]\displaystyle{ \quad \int{\mathbf{w_1^\dagger w_1}}\ dV = 1 \qquad }[/math]and [math]\displaystyle{ \quad \int{\mathbf{w_2^\dagger w_2}}\ dV = 1 }[/math] </equation>

It is now interesting to consider the movement of this system without outer influences, since the momentum must be conserved for the overall system. To take this into consideration, it is particularly convenient to use the common set of equations.

The movement is introduced according to the same principle as before, only now the operators which generate the movement must further be tracefree, such that the total momentum is zero. The properties of the generators of the movement are thus:

1. Each dimension has a linearly independent operator.
2. They are normalised and therefore length conserving for each part of the defects.
3. They have real (=Hermitian) eigenvalues, such that the momentum is observable.
4. They are altogether tracefree and thus conserve the total momentum.

In three dimensions, these properties are fulfilled by operators which can be constructed from Pauli matrices [math]\displaystyle{ \sigma^j }[/math]:

<equation id="eqn:su3.01" shownumber> [math]\displaystyle{ L_{\mathbf{m},\ defect,rel.} = \mathbf{\overline{W}_\alpha} \left( -\xi + 2\overset{\approx}{M} \cdot \sigma^j \cdot \partial_j\right)\mathbf{W_\alpha} }[/math] </equation>

Where [math]\displaystyle{ \sigma^1=\left(\begin{array}{cc} 0 & 1 \\ 1 & 0 \end{array}\right) }[/math], [math]\displaystyle{ \sigma^2=\left(\begin{array}{cc} 0 & -i \\ i & 0 \end{array}\right) }[/math] and [math]\displaystyle{ \sigma^3=\left(\begin{array}{cc} 1 & 0 \\ 0 & -1 \end{array}\right) }[/math].

This choice of Pauli matrices is appropriate for a defect pair which is separated on the z-axis. It can be seen that when there is movement in the x and y directions, the deformation fields mix, but not for the movement in the z direction.

Correspondingly, in four dimensions one needs a set of four linearly independent operators which are tracefree and observable. In the case of a flat observer, three of these operators are therefore hermitian and one anti-hermitian (due to the negative metric element). These properties are fulfilled by the Dirac matrices [math]\displaystyle{ \gamma^j }[/math] multiplied by [math]\displaystyle{ i }[/math]:

<equation id="eqn:su3.01" shownumber> [math]\displaystyle{ L_{\mathbf{m},\ defect,rel.} = \mathbf{\overline{W}_\alpha} \left( -\xi + 2\overset{\approx}{M} \cdot i \cdot \gamma^j \cdot \partial_j\right)\mathbf{W_\alpha} }[/math] </equation>

Where e.g [math]\displaystyle{ \gamma^0=\left(\begin{array}{cc} 1 & 0 \\ 0 & -1 \end{array}\right) }[/math], [math]\displaystyle{ \gamma^j=\left(\begin{array}{cc} 0 & \sigma^j \\ -\sigma^j & 0 \end{array}\right) }[/math] (in this notation, each element corresponds to a 2x2 matrix, [math]\displaystyle{ j=1,2,3 }[/math]).

 

Symmetry breaking

So far, an isotropic lattice was assumed. But, at least locally, this assumption does not hold true for elastic lattices with a finite elementary cell size. In an ordered crystal structure this is not even valid globally. Disordered lattices build an isotropic structure on average, but the isotropy is in any case broken locally. The concept of symmetry breaking is used in physics for phase transitions. With this in mind one can apply this concept by parametrising the measure for the breaking of the local isotropy (local [math]\displaystyle{ SO(3) }[/math] symmetry in three dimensions and local [math]\displaystyle{ SU(2) }[/math] symmetry in four dimensions).

Mathematically, the breaking of symmetry is very complex and will not be dealt with here in more detail (to this end, see e.g. , read in , orig. ). Instead, a brief and basic overview of the approach and the arising effects shall be given.

Approach:
A further potential is introduced which breaks the symmetry, then a valid solution is chosen from the emerging broken solutions, and the Lagrangian is expanded around it.

As a result one gets a new scalar field [math]\displaystyle{ H }[/math], which interacts with itself and the oscillations. With it further self-interactions of the oscillation modes arise.

Effects:
One of the main results of the symmetry breaking is that so called massive oscillation modes arise. These are damped exponentially and therefore show up only in the neighbourhood of the defects . These modes can be compared to the ones arising in the classical description of the elastic lattice with defect, which are also exponentially damped.

Chiral effects arise on top of that in specific defect types. Again, in the classical description, this quite likely corresponds to the distinction between defects whose strength is either greater or less than zero, where localised oscillations modes only arise in the case that [math]\displaystyle{ \xi\lt 0 }[/math] .

Both effects seem very similar in their descprition, yet their sameness would still have to be shown.

The mathematical terms, up to self-interactions, are presented in the following:

In summary, the total Lagrangian of a Frenkel defect is:

<equation id="eqn:su3.01" shownumber> [math]\displaystyle{ L_\mathbf{m}=L_{\mathbf{m},\ defect,rel.} + \ L_{\mathbf{m},\ inter.\ def.\leftrightarrow{}lattice} + L_{\mathbf{m},\ lattice} + L_{\mathbf{m},\ symm.break.} + L_{\mathbf{m},\ inter.-symm.break.} }[/math] </equation>

Where in the three-dimensional case one has:

<equation id="eqn:su3.01" shownumber> [math]\displaystyle{ L_{\mathbf{m},\ defect,rel.} = \mathbf{\overline{W}_\alpha} \left( -\xi + 2 \overset{\approx}{M} \cdot \sigma^j \cdot \partial_j \right) \mathbf{W_\alpha} }[/math] </equation>

<equation id="eqn:su3.01" shownumber> [math]\displaystyle{ \displaystyle L_{\mathbf{m},\ inter.\ def.\leftrightarrow{}lattice} = - \mathbf{\overline{W}_\alpha} \left( {\left[ 3 \overset{\approx}{K} \cdot \sigma^j v_j \right]}_{U(1)} + {\left[ \sum_{a=1,2,3} 3\overset{\approx}{K}_a^\prime \cdot \sigma^j {R_a v^a}_j \right]}_{SO(3)} \right) \mathbf{W_\alpha} }[/math] </equation>

<equation id="eqn:su3.01" shownumber> [math]\displaystyle{ \displaystyle L_{\mathbf{m},\ lattice} = - \overset{\approx}{\Phi}_0 \ - {\left[ \overset{\approx}{K} \cdot V_{ij} V^{ij} \right]}_{U(1)} - {\left[ \sum_{a=1,2,3} \overset{\approx}{K}_a^\prime \cdot V^{\prime a}_{ij} {V^{\prime a}}^{ij} \right]}_{SO(3)} }[/math] </equation>

<equation id="eqn:su3.01" shownumber> [math]\displaystyle{ L_{\mathbf{m},\ symm.break} = - \frac{1}{2} m_H^2 H^2 + \frac{1}{2} (\partial_i H)(\partial^i H) }[/math] </equation>

<equation id="eqn:su3.01" shownumber> [math]\displaystyle{ L_{\mathbf{m},\ inter.-symm.break} = o(H^3, \mathbf{v}^3) }[/math] </equation>

And in four dimensions with a flat observer:

<equation id="eqn:su3.01" shownumber> [math]\displaystyle{ L_{\mathbf{m},\ defect,rel.} = \mathbf{\overline{W}_\alpha} \left( -\xi + 2 \overset{\approx}{M} \cdot i \cdot \gamma^j \cdot \partial_j \right) \mathbf{W_\alpha} }[/math] </equation>

<equation id="eqn:su3.01" shownumber> [math]\displaystyle{ \displaystyle L_{\mathbf{m},\ inter.\ def.\leftrightarrow{}lattice} = - \mathbf{\overline{W}_\alpha} \left( {\left[ 4 \overset{\approx}{K} \cdot \gamma^j v_j \right]}_{U(1)} + {\left[ \sum_{a=1,2,3} 4\overset{\approx}{K}_a^\prime \cdot \gamma^j {\sigma_a v^a}_j \right]}_{SU(2)} \right) \mathbf{W_\alpha} }[/math] </equation>

<equation id="eqn:su3.01" shownumber> [math]\displaystyle{ \displaystyle L_{\mathbf{m},\ lattice} = - \overset{\approx}{\Phi}_0 \ - {\left[ \overset{\approx}{K} \cdot V_{ij} V^{ij} \right]}_{U(1)} - {\left[ \sum_{a=1,2,3} \overset{\approx}{K}_a^\prime \cdot V^{\prime a}_{ij} {V^{\prime a}}^{ij} \right]}_{SU(2)} }[/math] </equation>

<equation id="eqn:su3.01" shownumber> [math]\displaystyle{ L_{\mathbf{m},\ symm.break} = - \frac{1}{2} m_H^2 H^2 + \frac{1}{2} (\partial_i H)(\partial^i H) }[/math] </equation>

<equation id="eqn:su3.01" shownumber> [math]\displaystyle{ L_{\mathbf{m},\ inter.-symm.break} = o(H^3, \mathbf{v}^3) }[/math] </equation>

 

Open questions

This text gives an outline for the description of small defects in an elastic lattice. More particularly, possibilities for the inclusive description of movement and the advantages in the description of interaction by means of local gauge theories are presented. Nonetheless, many questions remain unanswered in all areas, and must be additionally examined in further work. A few are summarised here:

Lattice:

• Which effects arise from detailed lattice structures, e.g. from hexagonal or face-centerd-cubic lattices, or from lattices with a number of different masses?
• What does the transition to the macroscopic description (continuum limit) look like?
• Are there any further effects from external tensions or curved lattices?

Defect:

• Are there verifiable experiments for the presented mechanisms?
• Only simple point defects were considered. Yet in a lattice also line defects (dislocations) are possible. How would a treatment of such defects look like within the framework of the presented theory?

Movement:

• Is the description for the movement's mechanism correct?
• Is it correct to say that the potential of the movement doesn’t depend on the strength of the defect? What does its detailed explanation look like?

Symmetry breaking:

• What is the exact connection between local symmetry breaking and the localised modes in the traditional model of the linear lattice?
• What about the effects arising from a cutoff or from chirality?

 

References

 

<- Chapter 1

back to top

Chapter 3 ->

---

---

This work is licensed under a Creative Commons Attribution-ShareAlike 4.0 International License.